Statistical mechanics of fluids

Contents

Statistical mechanics of fluids#

General exprssion of probability distribution of fluids in phase space#

The probability of a state in a classical fluid system is $f (x^{N}, p^{N})$

f (x^{N}, p^{N}) = \frac{e^{- β H (x^{N} p^{N})}}{Z}

Momentum and position coordinates are separated thanks to the form of kinetic and potential energy terms

H (x^{N}, p^{N}) = K (p^{N}) + U (x^{N})

f (x^{N}, p^{N}) = Φ (p^{N}) P (r^{N})

Kinetic energy part giveus us Maxwell-Botlzman distribution or the ideal gas part of the partition function $Z_{p}$
Potential energy part gives us configruational parition function $Z_{x}$ evaluation of which is non-trivial and mostly can be done via simulaions:

Z (β, V, N) = Z_{p} \cdot Z_{x} = \frac{1}{λ^{3} N!} Q (β, V, N)

Q = \int d x^{N} e^{- β U (x^{N})}

Pressure is related to Free energy as

p = - \frac{\partial F}{\partial V} = \frac{\partial}{\partial V} l o g \int d x^{N} e^{- β U (x^{N})}

Volume dependence of partition function is in the integration limits! As volume grows, so does partition function. Therefore p is always positive. We can thus conclude that in equilibrium pressure is always a positive quantity

Reduced configruational distribution functions#

Hard fact: $P (r^{N})$ and Q do not factorize because of inteparticle interactions with stronger interactions implying stronger correlations in positions.
Probability of many-body systems: to find particle $1$ at $r_{1}$ , $2$ and $r_{2}$ …:

P (r^{N}) = P (r_{1}, r_{2}, . . . r_{N})

Marginal(ized) probability

ρ^{2 / N} (r_{1}, r_{2}) = \int d r_{3} . . . d r_{N} P (r_{1}, r_{2}, . . . r_{N})

Marginal(ized) probability for any particle 1 and 2

ρ^{2 / N} (r_{1}, r_{2}) = N (N - 1) \int d r_{3} . . . d r_{N} P (r_{1}, r_{2}, . . . r_{N})

Radial distribution function (RDF)#

For an isotropic fluid, we have one point probability density:

ρ^{1 / N} = N \frac{\int d r_{2} . . . d r_{N}}{\int d r_{1} . . . d r_{N}} = N \frac{V^{N - 2}}{V^{N}} = \frac{N}{V} = ρ

The joint distribution for an ideal gas:

ρ^{2 / N} = N (N - 1) \frac{\int d r_{3} . . . d r_{N}}{\int d r_{1} . . . d r_{N}} = N (N - 1) \frac{V^{N - 2}}{V^{N}} =\approx ρ^{2}

To measure the degree of spatial correlations, we introduce the Radial Distribution function (RDF):

g (r_{1}, r_{2}) = \frac{ρ^{2 / N} (r_{1}, r_{2})}{ρ^{2}}

RDF for isotropic fluids is due to translational invariance depends only on distance between particles:

g (r) = g (| r_{2} - r_{1} |)

Meaning of RDF

Since $ρ = ρ^{1 / N}$ , the conditional probability density of finding a particle at r distance away from a tagged particle at the origin is:

\frac{ρ^{2 / N} (0, r)}{ρ} = ρ g (r)

$ρ g (r)$ is then average density of particles at distance r given that a tagged particle is at the origin

Coordination shells and structure in fluids#

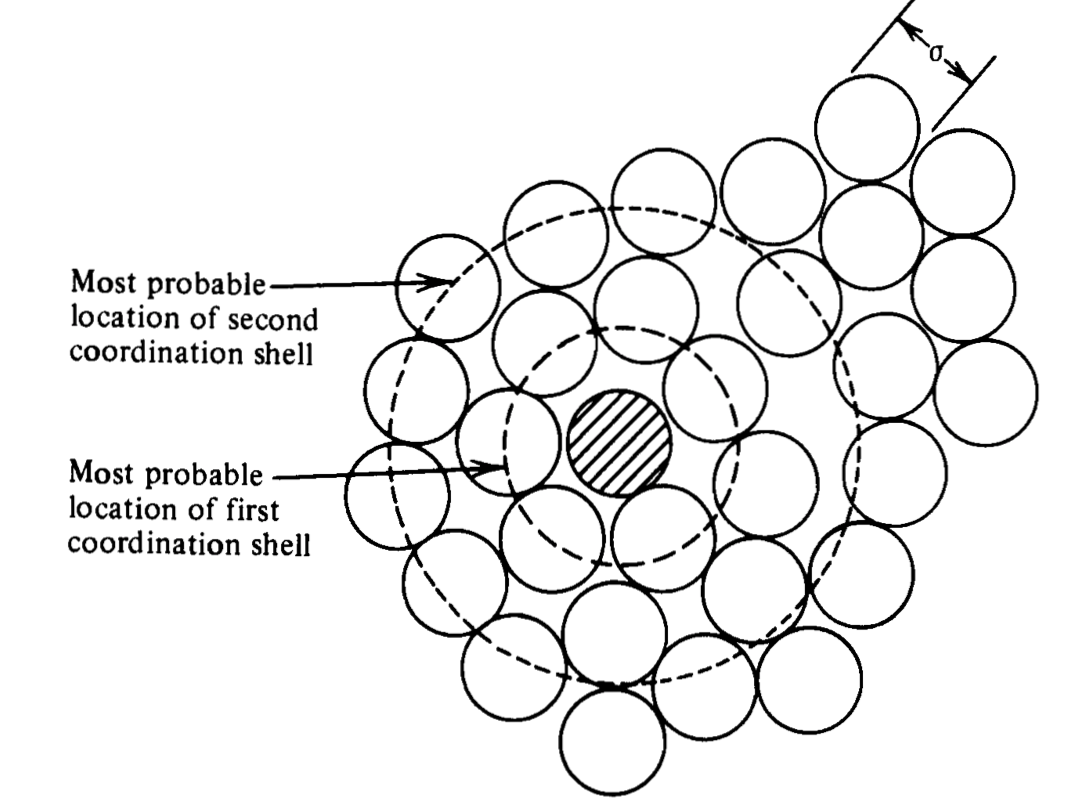
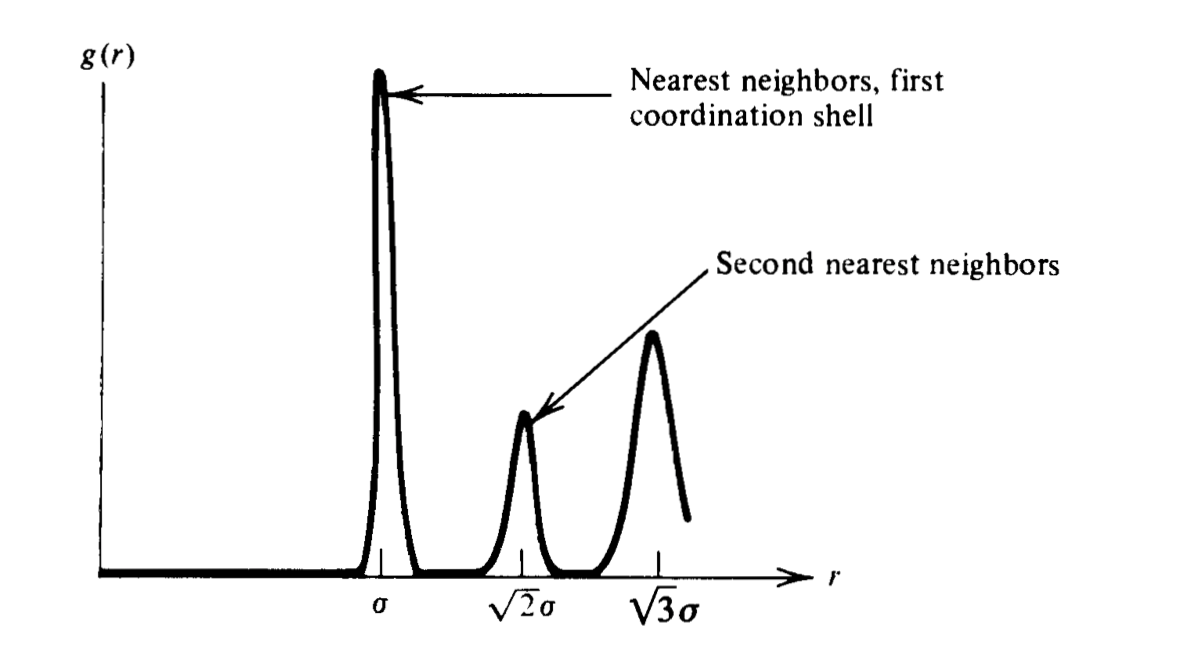
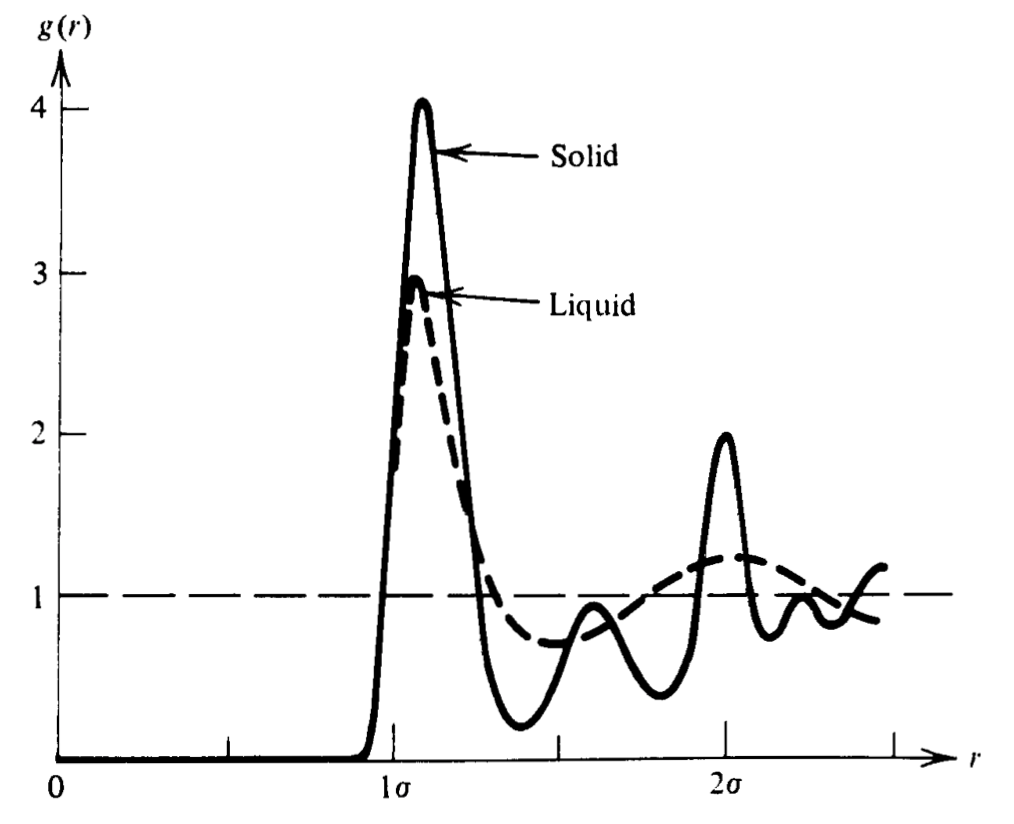
Reversible work theorem and potential of mean force#

g (r) = e^{- β w (r)}

w (r) = - β^{- 1} l o g [g (r)]

$w (r)$ Reversible work to bring two particles from infinity to distance r
$g (r)$ Radial distribution function

⟨ - \frac{d U (r^{N})}{d r_{1}} ⟩_{r_{1}, r_{2}} = - \frac{\int d r_{3} . . . d r_{N} \frac{d U (r^{N})}{d r_{1}} e^{- β U}}{\int d r_{3} . . . r_{N} e^{- β U}} = \frac{β^{- 1} \frac{d}{d r_{1}} \int d r_{3} . . . d r_{N} e^{- β U}}{\int d r_{3} . . . d r_{N} e^{- β U}} = β^{- 1} \frac{d}{d r_{1}} l o g \int d r_{3} . . . d r_{N} e^{- β U}

⟨ - \frac{d U (r^{N})}{d r_{1}} ⟩_{r_{1}, r_{2}} = β^{- 1} \frac{d}{d r_{1}} l o g N (N - 1) \int d r_{3} . . . d r_{N} e^{- β U} = β^{- 1} g (r_{1}, r_{2})

Thermodynamic properites of $g (r)$ #

⟨ E ⟩ = N ⟨ \frac{p^{2}}{2 m} ⟩ + ⟨ \sum_{j > i} u (| r_{i} - r_{j} |) ⟩

E / N = \frac{3}{2} k_{B} T + \frac{1}{2} ρ \int d r g (r) u (r)

Low density approximation for $g (r)$ #

w (r) = u (r) + Δ w (r)

g (r) = e^{- β u (r)} (1 + O (ρ))

Density expanesion and virial coefficients#

β p = ρ + B_{2} (T) ρ^{2} + O (ρ^{3})

B_{2} (T) = - \frac{1}{2} \int d r (e^{- β u (r)} - 1)