The need for quantization#
What you need to know
The energies of atoms, molecules, and light are quantized. This means that energy can only assume specific, discrete values rather than a continuous range.
Energy quantization is a fundamental principle of our physical reality, consistently observed in quantum experiments. Quantum mechanics provides a comprehensive explanation and accurate predictions of this phenomenon.
Key historical developments leading to the emergence of quantum mechanics include black body radiation, the double slit experiment, and the photoelectric effect.
Several macroscopic phenomena, such as the red glow of hot metals, the heat capacity of solids at low temperatures, and the colors of materials, are all manifestations of quantum effects.
What is the nature of light?#
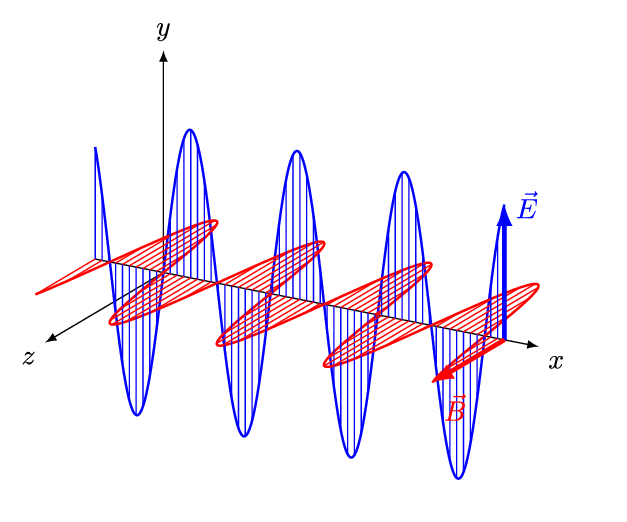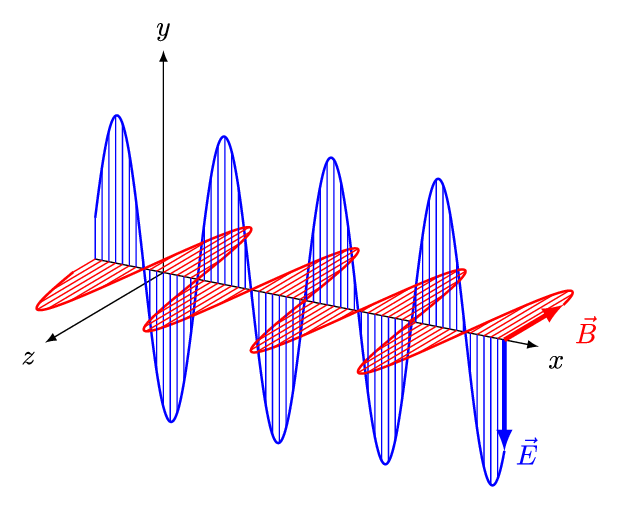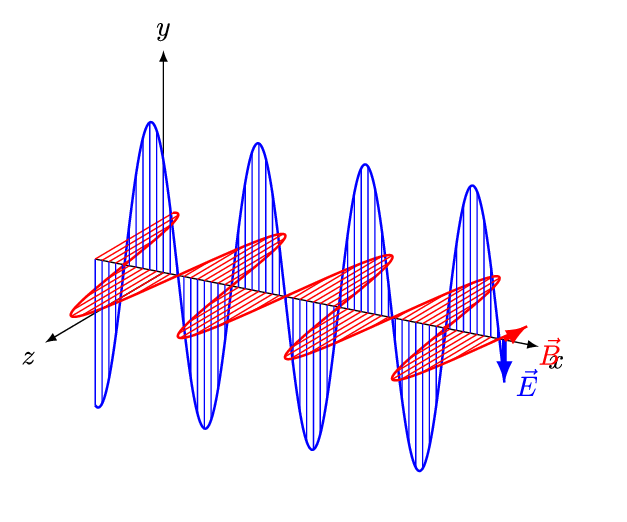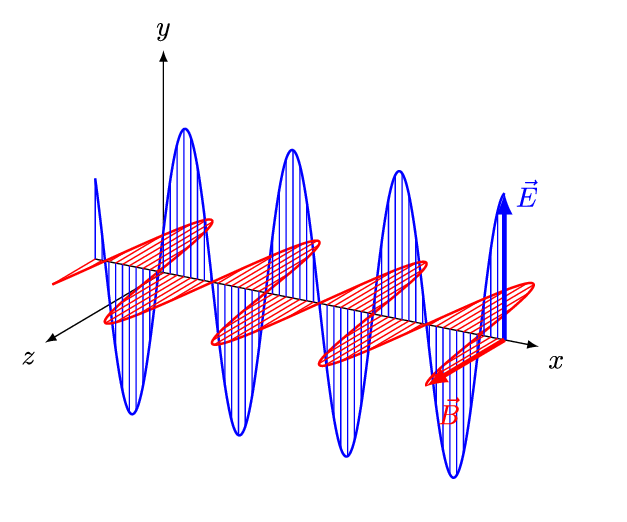
Fig. 7 Electromagneitc radiation has perpendicular electric and magnetic components which propogate with a speed of light. Unlike other forms of waves (water, sounds) light needs to medium and can travel in vaccum!#
According to the classical wave theory light is seen as a traveling wave consisting of electric and magnetic components.
We will soon see that this picture of light as enelectromagnetic wave is not the whole story and radically new ideas are needed to understand wide variety of phenomena invlving interaction of light with atoms and molecules.
Spectrum of electromagnetic waves#
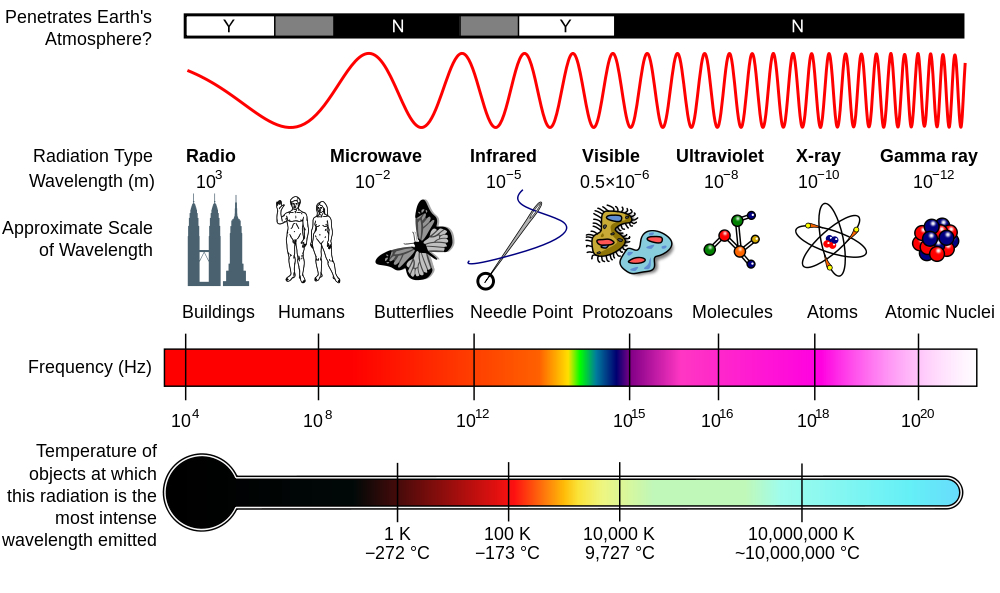
Fig. 8 Spectrum of Electromagneitc waves showing wavelengths and radiation type. Also shown are objects with comparable size as the wavelength. Also shown are temperatures of objects which radiate different wavelengths. You can see a clear link betwen how “hot” and how much energy the radiation contains.#
The visible light. occupies a narrow frequency region in between.
High-frequency waves carry much higher energy. This means X-ray or Gamma-rays can only be generated by heating “stuff” up at very high temperatures. This happens naturally at the core of the sun!
Low-frequency waves carry less energy. Can be generated in a “microwave” or by broadcasting antennas.
So what is the relationship between frequency of radiation
Relationship between frequency, wavelength and speed of light.#
Fig. 9 Definitions of wavelength
Wavelength, frequency and speed of light
Speed of light in vacuum,
Frequency of
Wavelength of
Black body as a model for heated objects.#
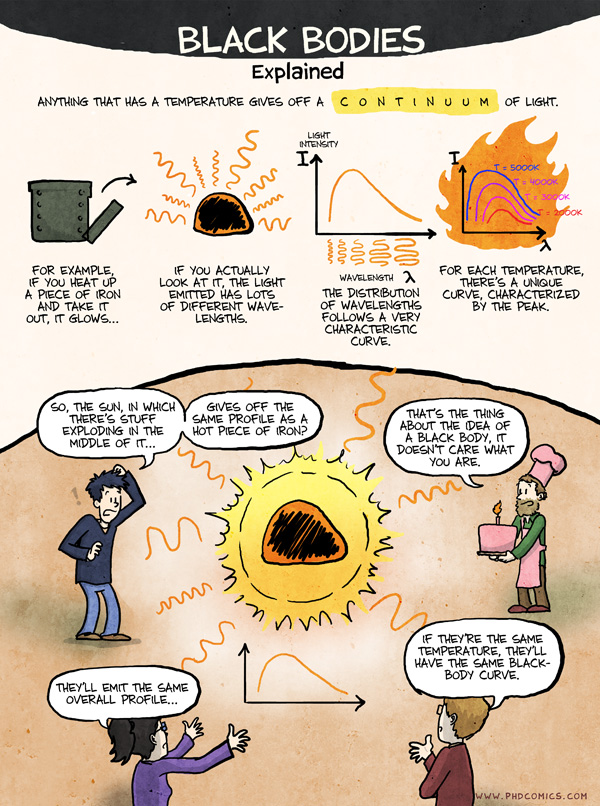
Fig. 10 Black body radiation guide from PhDcomics!#
Watch this beautiful animation where the first 3 minutes is solely focused on blackbody radiation
Black body as an idealized model#
Definition of black body
A black body is a model, an idealized system just like an ideal gas model in thermodynamics. Black body is assumed to be in thermodynamic equilibrium, maintained at some constant Temperature T which both absorbs and emits every wavelength of electromagnetic radiation
Equilibrium condition in thermodynamics means that the system emits as much energy as it absorbs. In other words outflux = influx.
The reason its called black body is that it absorbs every wavelength that hits the surface, therefore, appearing as 100% perfect black object.
If an object has a color, it is because it is reflecting certain wavelengths of light which then gets detected by our eye retina. The distribution of wavelengths, which is emitted by a blackbody, is determined only by its temperature!
Fig. 11 When heating up a material we observe three things. 1. Radiation intensity of material increases, implying higher radiation energy. 2. Distribution of wavelengths emitted shifts to lower values. Or we can say the distribution of frequency shifts to higher values. 3. The color of the material changes from red to yellow to blue.#
Ultraviolet catastrophe of classical mechanics#
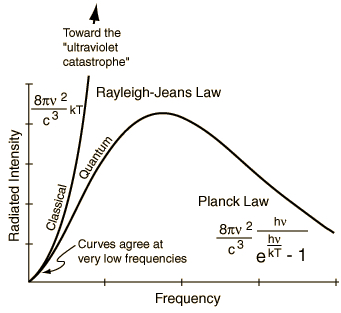
Fig. 12 Predictions of CM and QM diverge in the high frequency (low wavelength) limit where CM predicts infinite energy and QM predicts insufficent thermal energy for radiation.#
What is radiation in classical mechanics? Radiation is considered a wave with frequency
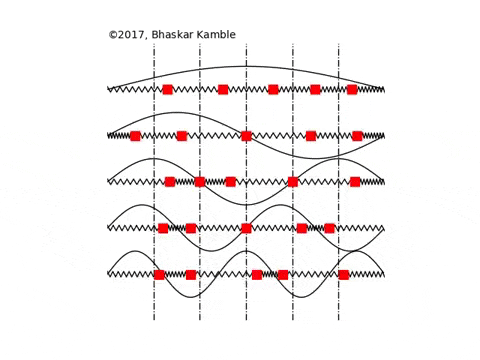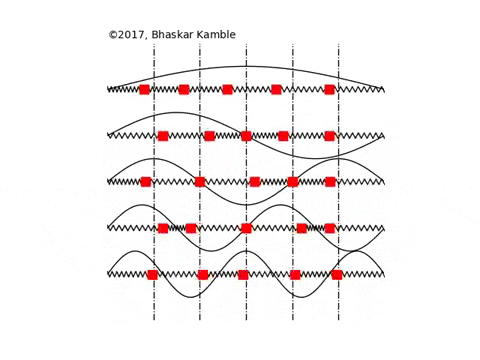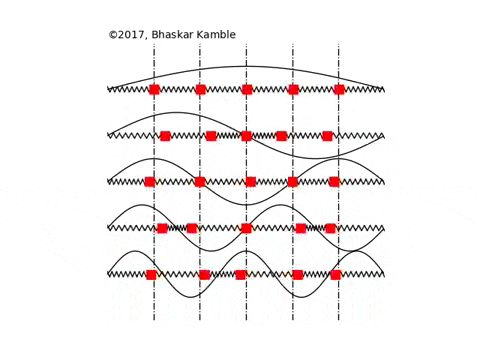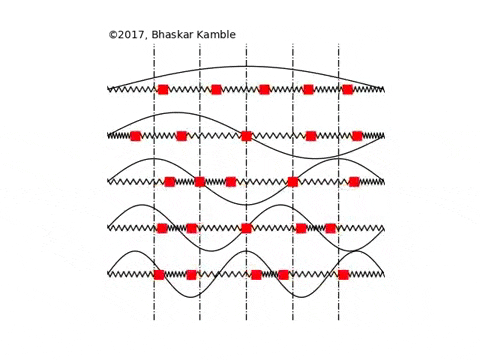
Fig. 13 Visualuzation of atomic vibrations in a solid body. These vibrational modes are called phonons not to be confused with photons to be introduced in next section!#
Packing wave modes in a box One can fit more of high fequency (short wavelength) waves in the box than small frequency ones. The number of waves we can fit in a cubic box in the frequency region
Equipartition of energy From thermodynamics we know that in equilibrium each degree of freedom or each oscillator gets the same
Every vibrating spring in heated body thus has same energy regardless of frequency, think about this assumption for a sec!
Radiation Energy Distribution: Distribution of radiated frequencies is then product of average thermal energy and number of springs in a frequency interval
Ultraviolet catastrophe: Energy distribution shoots to infinity at high
Quiz
What is the problem with classical mechanical explanation of black body radiation?
It is not corectly applied.
CM makes some uncontrolled approximations about energy.
CM can not model waves.
Classical mechanics does not have a way to account for the quantization of energy.
Max Planck and the trick of quantization#
In 1900 Planck found that the theoretical curve can very closely match the experimental curve if one postulates that only discrete (quantized) values of energy are possible.
Plack equation
Unit of Planck’s constant,
Unit of frequency
Unit of Energy
This means atoms and molecules absorb and emit radiation in discrete quantities, multiples of
When light is emitted or absorbed, the atom or molecule jumps from one state to another and the energy difference
Note how small
The black body radiation distribution function#
Deriving Black Body radiation formula
Planck hypothesized that the energy of oscillators in a black body is quantized and given by:
where
The average energy of an oscillator is found by summing over all possible energies, weighted by the Boltzmann factor:
Substituting
This sum is a geometric series. For the geometric series of the form:
The sum is given by:
In the context of Planck’s derivation, we use the series:
This series can be summed as:
The series involving
This can be evaluated using the derivative with respect to
Substituting
Using these results, Planck’s formula for the average energy becomes:
The energy density
This is Planck’s law, which describes the spectral density of radiation emitted by a black body in thermal equilibrium at a temperature
Assuming that the energy of an oscillator is quantized, Planck dervied a new expression for average energy which now depends on frequency of oscillations unlike classical expression of
With this expression we end up with a distirbution of oscillator energies which tends to zero in the high frequency limit.
You can also express distribution in terms of wavelength by making the subsittion
The expressions for
In some books you may also find black body radiation characterized via the radiation flux which is a radiation measured per unit wavelength and per unit solid angle
Wien’s displacement law#
Connecting temperature of black body with wavelength or frequency. The energy density peaks at a wavelength
Explore black body radiation#
Applications of Black Body radiation#
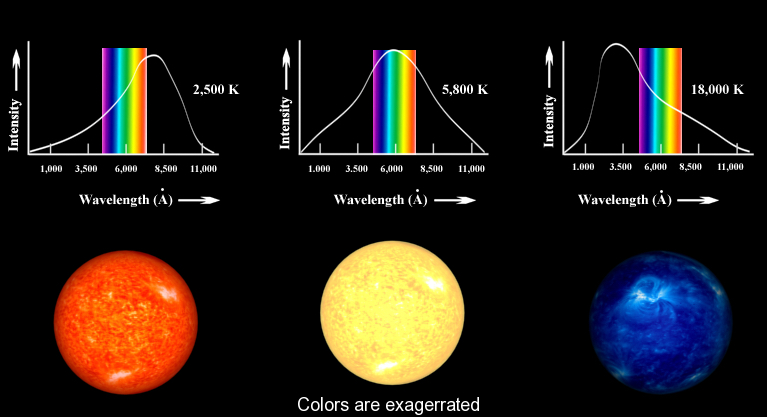
Fig. 14 The black body is used as a standard with which the absorption of real bodies is compared. To a good approximation, stars radiate like blackbody radiators. Thus we can use blackbody radiation as a model to infer the temperature of the stars from their colors! Find out more in this video on Visible Light Waves#
Problems#
Problem 1#
Calculating the Wavelength of a Photon Using Planck’s Equation
A photon is emitted with an energy of
Planck’s constant,
Speed of light,
Solution:
First, convert the energy of the photon from electron volts (eV) to joules (J):
Now, use Planck’s equation to relate the energy
Rearranging to solve for
Substitute the known values:
The wavelength of the photon is approximately
Problem 2#
Question: Wien’s displacement law
Using Wien’s displacement law, determine the wavelength
Solution:
Wien’s displacement law states that the wavelength at which the spectral radiance of a blackbody peaks is inversely proportional to the temperature:
where
For
So, the peak wavelength