BO approximation#
What you need to know
Born-Oppenheimer (BO) approximation, Because nuclei are much heavier than electrons, the Schrodinger equation can be approximately separated into the nuclear and the electron parts. Thus the electronic Schrodinger equation for a molecule can be solved separately at each fixed nuclear configuration. This is called the Born-Oppenheimer approximation.
BO approximation allows us to extend multi-electron treatment of atoms to molecules.
Single electron wavefunctions of molecules are called Molecular Orbitals (MO)
Geometry of
Nuclear geometry is a varyable parameter in the problem: For different values of R one gets different energies and MOs.
Simplest molecule#
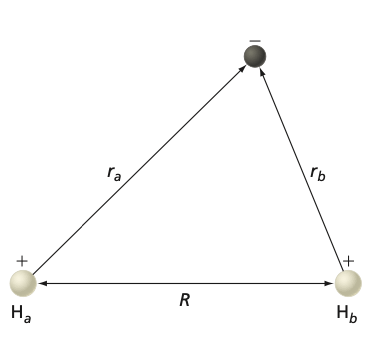
Fig. 98 Coordinates used to describe
In the following, we will consider the simplest molecule
where
where
Note that the Hamiltonian includes also the quantum mechanical kinetic energy for the protons. As such the wavefunction depends on
The BO approximation#
Fig. 99 Max Born#
Fig. 100 Robert J Oppenheimer#
Because the nuclear mass
where
Note that equation depends parametrically on
Because